Home /
Expert Answers /
Physics /
consider-a-spherically-symmetric-charge-distribution-with-a-total-charge-q-and-charge-density-rho-pa717
(Solved): Consider a spherically symmetric charge distribution with a total charge Q and charge density \rho = ...
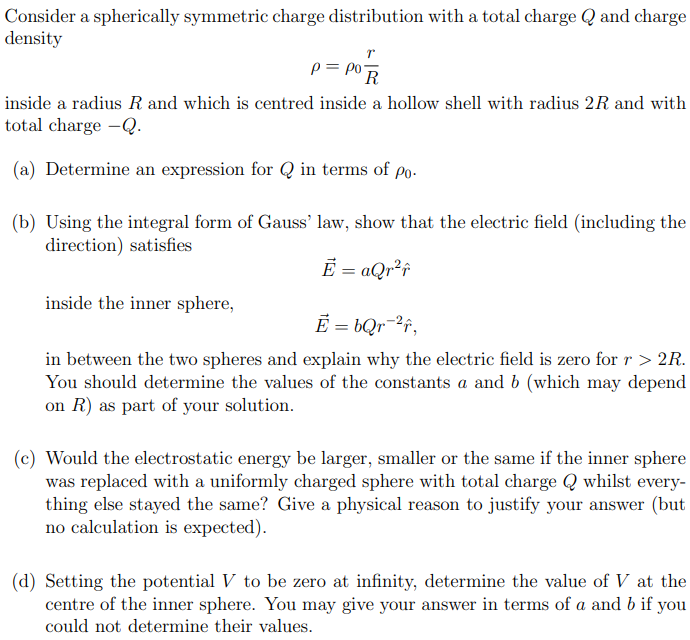
Consider a spherically symmetric charge distribution with a total charge Q and charge
density
\rho =\rho _(0)(r)/(R)
inside a radius R and which is centred inside a hollow shell with radius 2R and with
total charge -Q.
(a) Determine an expression for Q in terms of \rho _(0).
(b) Using the integral form of Gauss' law, show that the electric field (including the
direction) satisfies
vec(E)=aQr^(2)hat(r)
inside the inner sphere,
vec(E)=bQr^(-2)hat(r),
in between the two spheres and explain why the electric field is zero for r>2R.
You should determine the values of the constants a and bR Q whilst every-
thing else stayed the same? Give a physical reason to justify your answer (but
no calculation is expected).
(d) Setting the potential V to be zero at infinity, determine the value of V at the
centre of the inner sphere. You may give your answer in terms of a and b if you
could not determine their values.